In this article, we study the asymptotic behavior of solutions to the delay differential equation
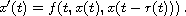
It is shown that every solution tends to either
 or a constant as
or a constant as
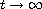 .
.
Qiyuan Zhou
Abstract:
In this article, we study the asymptotic behavior of solutions
to the delay differential equation
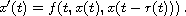
It is shown that every solution tends to either
 or a constant as
or a constant as
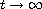 .
.
Submitted May 20, 2011. Published August 10, 2011.
Math Subject Classifications: 34C12, 39A11.
Key Words: Asymptotic behavior; delay differential equation
Show me the PDF file (207 KB), TEX file, and other files for this article.
 |
Qiyuan Zhou College of Mathematics and Computer Science Hunan University of Arts and Science Changde, Hunan 415000, China email: zhouqiyuan65@yahoo.com.cn |
|---|
Return to the EJDE web page