Using the Leray Schauder nonlinear alternative, we prove the existence of a nontrivial solution for the three-point boundary-value problem

where
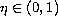 ,
,
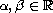 ,
,
![$f\in C([0,1] \times\mathbb{R},\mathbb{R})$](gifs/ad.gif) .
Some examples are given to illustrate the results obtained.
.
Some examples are given to illustrate the results obtained.
Assia Guezane-Lakoud, Smail Kelaiaia
Abstract:
Using the Leray Schauder nonlinear alternative, we prove the
existence of a nontrivial solution for the three-point
boundary-value problem

where
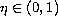 ,
,
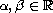 ,
,
![$f\in C([0,1] \times\mathbb{R},\mathbb{R})$](gifs/ad.gif) .
Some examples are given to illustrate the results obtained.
.
Some examples are given to illustrate the results obtained.
Submitted March 19, 2010. Published September 27, 2010.
Math Subject Classifications: 34B10, 34B15.
Key Words: Fixed point theorem; three-point boundary-value problem;
non trivial solution.
Show me the PDF file (203 KB), TEX file, and other files for this article.
 |
Assia Guezane-Lakoud Department of Mathematics, Faculty of Sciences University Badji Mokhtar, B.P. 12, 23000, Annaba, Algeria email: a_guezane@yahoo.fr |
|---|---|
| Smail Kelaiaia Department of Mathematics, Faculty of Sciences University Badji Mokhtar, B.P. 12, 23000, Annaba, Algeria email: kelaiaiasmail@yahoo.fr |
Return to the EJDE web page