By an application of Bonanno's three critical point theorem, we establish the existence of a nontrivial solution to the problem
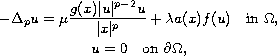
under some restrictions on g,a and f for certain positive values of
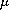 and
and
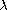 .
.
Jagmohan Tyagi
Abstract:
By an application of Bonanno's three critical point theorem,
we establish the existence of a nontrivial solution to the problem
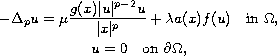
under some restrictions on g,a and f for certain positive
values of
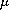 and
and
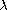 .
.
Submitted April 15, 2010. Published August 20, 2010.
Math Subject Classifications: 35J92, 35J75.
Key Words: p-Laplacian; singularity; multiple solutions.
Show me the PDF file (240 KB), TEX file, and other files for this article.
 |
Jagmohan Tyagi TIFR Centre For Applicable Mathematics Post Bag No.-6503, Sharda Nagar Chikkabommasandra, Bangalore-560065, Karnataka, India email: jagmohan.iitk@gmail.com, jtyagi1@gmail.com |
|---|
Return to the EJDE web page