Electron. J. Diff. Equ.,
Vol. 2010(2010), No. 110, pp. 1-19.
Effect of hyperviscosity on the Navier-Stokes turbulence
Abdelhafid Younsi
Abstract:
In this article, we modified the Navier-Stokes equations by
adding a higher order artificial viscosity term to the conventional
system. We first show that the solution of the regularized system
converges strongly to the solution of the conventional system as
the regularization parameter approaches zero, for each dimension
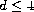 .
Then we show that the use of this artificial viscosity
term leads to truncated the number of degrees of freedom in the
long-time behavior of the solutions to these equations.
This result suggests that the hyperviscous Navier-Stokes system
is an interesting model for three-dimensional fluid turbulence.
.
Then we show that the use of this artificial viscosity
term leads to truncated the number of degrees of freedom in the
long-time behavior of the solutions to these equations.
This result suggests that the hyperviscous Navier-Stokes system
is an interesting model for three-dimensional fluid turbulence.
Submitted December 2, 2009. Published August 9, 2010.
Math Subject Classifications: 76D05, 76F20, 35B30, 35B41, 35B65, 37L30, 37K40.
Key Words: Navier-Stokes equations; hyperviscosity;
weak solutions; attractor dimension; turbulence models
An addendum was attached on September 27, 2011. It corrects some misprints
and presents another proof of estimates for the dimension of the attractor.
Show me the PDF file (309 KB),
TEX file, and other files for this article.
 |
Abdelhafid Younsi
Faculty of Mathematics USTHB
BP32 EL ALIA16111 Algiers, Algeria
email: younsihafid@gmail.com
|
|---|
Return to the EJDE web page
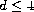 .
Then we show that the use of this artificial viscosity
term leads to truncated the number of degrees of freedom in the
long-time behavior of the solutions to these equations.
This result suggests that the hyperviscous Navier-Stokes system
is an interesting model for three-dimensional fluid turbulence.
.
Then we show that the use of this artificial viscosity
term leads to truncated the number of degrees of freedom in the
long-time behavior of the solutions to these equations.
This result suggests that the hyperviscous Navier-Stokes system
is an interesting model for three-dimensional fluid turbulence.
