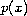Electron. J. Diff. Eqns., Vol. 2009(2009), No. 72, pp. 1-12.

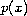Existence of solutions for a $p(x)$-Laplacian non-homogeneous equations

Ionica Andrei

Abstract:
We study the boundary value problem
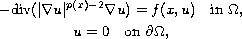
$$\displaylines{ -\hbox{\rm div}(|\nabla u|^{p(x)-2}\nabla u)=f(x,u)\quad \hbox{in }\Omega, \cr u=0\quad \hbox{on }\partial \Omega, }$$
where 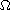$\Omega$ is a smooth bounded domain in 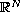$\mathbb{R}^N$. Our attention is focused on the cases when
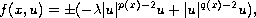
$$ f(x,u)=\pm (-\lambda |u|^{p(x)-2}u+|u|^{q(x)-2}u), $$
where 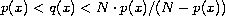$ p(x)<q(x)<N\cdot p(x)/(N-p(x))$ for $x$ in $\Omega$.

Submitted March 9, 2009. Published June 2, 2009.
Math Subject Classifications: 35D05, 35J60, 58E05
Key Words: p(x)-Laplace operator; generalized Lebesgue-Sobolev space; critical point; weak solution.

Show me the PDF file (238 KB), TEX file, and other files for this article.

Ionica Andrei
Department of Mathematics, High School of Cujmir
227150 Cujmir, Romania
email: andreiionica2003@yahoo.com

Return to the EJDE web page