Michinori Ishiwata, Takayoshi Ogawa, Futoshi Takahashi
Abstract:
We consider the following nonlinearly perturbed version
of the elliptic-parabolic system of Keller-Segel type:
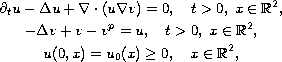
where
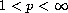 .
It has already been shown that the
system admits a positive solution
for a small nonnegative initial data in
.
It has already been shown that the
system admits a positive solution
for a small nonnegative initial data in
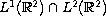 which corresponds to the local minimum of the associated energy
functional to the elliptic part of the system.
In this paper, we show that for a radially symmetric nonnegative
initial data, there exists another positive solution which
corresponds to the critical point of mountain-pass type.
The
which corresponds to the local minimum of the associated energy
functional to the elliptic part of the system.
In this paper, we show that for a radially symmetric nonnegative
initial data, there exists another positive solution which
corresponds to the critical point of mountain-pass type.
The
 -component
of the solution bifurcates from the unique
positive radially symmetric solution of
-component
of the solution bifurcates from the unique
positive radially symmetric solution of
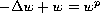 in
in
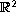 .
.
Submitted August 22, 2008. Published February 16, 2009.
Math Subject Classifications: 35K15, 35K55, 35Q60, 78A35.
Key Words: Multiple existence; elliptic-parabolic system;
unconditional uniqueness.
Show me the PDF file (250 KB), TEX file, and other files for this article.
 |
Michinori Ishiwata Common Subject Division, Muroran Institute of Technology Muroran 050-8585, Japan email: ishiwata@mmm.muroran-it.ac.jp |
|---|---|
 |
Takayoshi Ogawa Mathematical Institute, Tohoku University Sendai 980-8578, Japan email: ogawa@math.tohoku.ac.jp |
 |
Futoshi Takahashi Graduate School of Science, Osaka City University Osaka 558-8585, Japan email: futoshi@sci.osaka-cu.ac.jp |
Return to the EJDE web page