Electron. J. Diff. Equ.,
Vol. 2009(2009), No. 163, pp. 1-9.
Three positive solutions for a system of singular generalized
Lidstone problems
Jiafa Xu, Zhilin Yang
Abstract:
In this article, we show the existence of at least three positive
solutions for the system of singular generalized Lidstone boundary
value problems
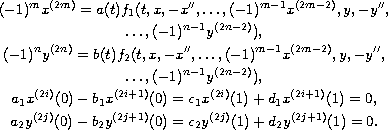
The proofs of our main results are based on the Leggett-Williams
fixed point theorem. Also, we give an example to illustrate our
results.
Submitted October 7, 2009. Published December 21, 2009.
Math Subject Classifications: 34A34, 34B18, 45G15, 47H10.
Key Words: Singular generalized Lidstone problem; positive solution;
cone; concave functional.
Show me the PDF file (192 KB),
TEX file, and other files for this article.
 |
Jiafa Xu
Department of Mathematics, Qingdao Technological University
No 11, Fushun Road, Qingdao, China
email: xujiafa292@sina.com |
|---|
 |
Zhilin Yang
Department of Mathematics, Qingdao Technological University
No 11, Fushun Road, Qingdao, China
email: zhilinyang@sina.com |
|---|
Return to the EJDE web page