Electron. J. Diff. Eqns.,
Vol. 2009(2009), No. 04, pp. 1-14.
Annulus oscillation criteria for second order
nonlinear elliptic differential equations with damping
Rong-Kun Zhuang
Abstract:
We establish oscillation criteria for the second-order
elliptic differential equation
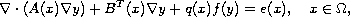
where
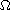 is an exterior domain in
is an exterior domain in
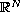 .
These
criteria are different from most known ones in the sense that they
are based on the information only on a sequence of annulus
of
.
These
criteria are different from most known ones in the sense that they
are based on the information only on a sequence of annulus
of
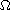 ,
rather than on the whole exterior domain
,
rather than on the whole exterior domain
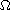 .
Both the cases when
.
Both the cases when
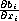 exists
for all i and when it does not exist for some i
are considered.
exists
for all i and when it does not exist for some i
are considered.
Submitted May 28, 2008. Published January 2, 2009.
Math Subject Classifications: 35J60, 34C10.
Key Words: Nonlinear elliptic differential equation; second order;
oscillation; annulus criteria.
Show me the
PDF file (263 KB),
TEX file, and other files for this article.
 |
Rong-Kun Zhuang
Department of Mathematics, Huizhou University
Huizhou 516015, China
Department of Mathematics, Sun Yat-sen University
Guangzhou 510275, China
email: rkzhuang@163.com
|
|---|
Return to the EJDE web page
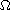 is an exterior domain in
is an exterior domain in
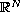 .
These
criteria are different from most known ones in the sense that they
are based on the information only on a sequence of annulus
of
.
These
criteria are different from most known ones in the sense that they
are based on the information only on a sequence of annulus
of
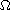 ,
rather than on the whole exterior domain
,
rather than on the whole exterior domain
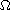 .
Both the cases when
.
Both the cases when
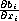 exists
for all i and when it does not exist for some i
are considered.
exists
for all i and when it does not exist for some i
are considered.
