Electron. J. Diff. Eqns.,
Vol. 2008(2008), No. 67, pp. 1-9.
Impulsive dynamic equations on a time scale
Eric R. Kaufmann, Nickolai Kosmatov, Youssef N. Raffoul
Abstract:
Let
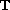 be a time scale such that
be a time scale such that
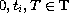 ,
,
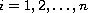 ,
and
,
and
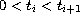 . Assume each
. Assume each
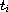 is dense.
Using a fixed point theorem due to Krasnosel'ski\i}, we show that the
impulsive dynamic equation
is dense.
Using a fixed point theorem due to Krasnosel'ski\i}, we show that the
impulsive dynamic equation
![$$\displaylines{
y^{\Delta}(t) = -a(t)y^{\sigma}(t)+ f ( t, y(t) ),\quad t \in (0, T],\cr
y(0) = 0,\cr
y(t_i^+) = y(t_i^-) + I (t_i, y(t_i) ), \quad i = 1, 2, \dots, n,
}$$](gifs/af.gif)
where
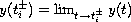 , and
, and
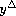 is
the
is
the
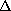 -derivative
on
-derivative
on
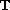 ,
has a solution.
Under a slightly more stringent inequality we show that the solution
is unique using the contraction mapping principle. Finally, with the
aid of the contraction mapping principle we study the stability of
the zero solution on an unbounded time scale.
,
has a solution.
Under a slightly more stringent inequality we show that the solution
is unique using the contraction mapping principle. Finally, with the
aid of the contraction mapping principle we study the stability of
the zero solution on an unbounded time scale.
Submitted November 20, 2007. Published May 1, 2008.
Math Subject Classifications: 34A37, 34A12, 39A05.
Key Words: Fixed point theory; nonlinear dynamic equation;
stability; impulses.
Show me the
PDF file (226 KB),
TEX file, and other files for this article.
 |
Eric R. Kaufmann
Department of Mathematics and Statistics
University of Arkansas at Little Rock
Little Rock, Arkansas 72204-1099, USA
email: erkaufmann@ualr.edu
|
|---|
 |
Nickolai Kosmatov
Department of Mathematics and Statistics
University of Arkansas at Little Rock
Little Rock, Arkansas 72204-1099, USA
email: nxkosmatov@ualr.edu
|
|---|
 |
Youssef N. Raffoul
Department of Mathematics,
University of Dayton
Dayton, OH 45469-2316, USA
e-mail: youssef.raffoul@notes.udayton.edu |
|---|
Return to the EJDE web page
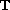 be a time scale such that
be a time scale such that
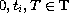 ,
,
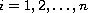 ,
and
,
and
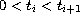 . Assume each
. Assume each
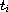 is dense.
Using a fixed point theorem due to Krasnosel'ski\i}, we show that the
impulsive dynamic equation
is dense.
Using a fixed point theorem due to Krasnosel'ski\i}, we show that the
impulsive dynamic equation
![$$\displaylines{
y^{\Delta}(t) = -a(t)y^{\sigma}(t)+ f ( t, y(t) ),\quad t \in (0, T],\cr
y(0) = 0,\cr
y(t_i^+) = y(t_i^-) + I (t_i, y(t_i) ), \quad i = 1, 2, \dots, n,
}$$](gifs/af.gif)
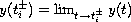 , and
, and
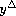 is
the
is
the
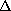 -derivative
on
-derivative
on
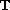 ,
has a solution.
Under a slightly more stringent inequality we show that the solution
is unique using the contraction mapping principle. Finally, with the
aid of the contraction mapping principle we study the stability of
the zero solution on an unbounded time scale.
,
has a solution.
Under a slightly more stringent inequality we show that the solution
is unique using the contraction mapping principle. Finally, with the
aid of the contraction mapping principle we study the stability of
the zero solution on an unbounded time scale.


