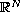 with periodic coefficients
with periodic coefficients
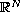 with periodic coefficients
with periodic coefficients
Ruy C. Charao, Eleni Bisognin, Vanilde Bisognin, Ademir F. Pazoto
Abstract:
In this work we study the asymptotic behavior of solutions of a
dissipative plate equation in
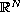 with periodic
coefficients. We use the Bloch waves decomposition and a
convenient Lyapunov function to derive a complete asymptotic
expansion of solutions as
with periodic
coefficients. We use the Bloch waves decomposition and a
convenient Lyapunov function to derive a complete asymptotic
expansion of solutions as
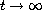 . In a first
approximation, we prove that the solutions for the linear model
behave as the homogenized heat kernel.
. In a first
approximation, we prove that the solutions for the linear model
behave as the homogenized heat kernel.
Submitted June 7, 2007. Published March 29, 2008.
Math Subject Classifications: 35C20, 35B40, 35B27, 35L15.
Key Words: Asymptotic behavior; homogenization;
partial differential equations; media with periodic structure;
second-order hyperbolic equations.
Show me the PDF file (355 KB), TEX file, and other files for this article.
| Ruy Coimbra Charão Departamento de Matemática, Universidade Federal de Santa Catarina P. O. Box 476, CEP 88040-900, Florianópolis, SC, Brasil email: charao@mtm.ufsc.br | |
| Eleni Bisognin Centro Universitário Franciscano Campus Universitário, 97010-032, Santa Maria, RS, Brasil email: eleni@unifra.br | |
| Vanilde Bisognin Centro Universitário Franciscano Campus Universitário, 97010-032, Santa Maria, RS, Brasil email: vanilde@unifra.br | |
 |
Ademir Fernando Pazoto Instituto de Matemática, Universidade Federal do Rio de Janeiro P. O. Box 68530, CEP 21945-970, Rio de Janeiro, RJ, Brasil email: ademir@acd.ufrj.br |
Return to the EJDE web page