We study the Cauchy problem associated with the nonlinear elliptic - parabolic equation
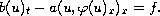
We prove an
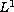 -contraction
principle and hence the uniqueness of
entropy solutions, under rather general assumptions on the data.
-contraction
principle and hence the uniqueness of
entropy solutions, under rather general assumptions on the data.
Stanislas Ouaro, Hamidou Toure
Abstract:
We study the Cauchy problem associated with the nonlinear
elliptic - parabolic equation
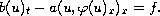
We prove an
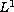 -contraction
principle and hence the uniqueness of
entropy solutions, under rather general assumptions on the data.
-contraction
principle and hence the uniqueness of
entropy solutions, under rather general assumptions on the data.
Submitted November 11, 2005. Published May 29, 2007.
Math Subject Classifications: 35K65, 35L65.
Key Words: Elliptic; parabolic; degenerate; weak solution;
entropy solution; L1-contraction principle.
Show me the PDF file (244K), TEX file, and other files for this article.
 |
Stanislas Ouaro Laboratoire d'Analyse Mathématique des Equations (LAME), UFR. Sciences Exactes et Appliquées, Université de Ouagadougou 03 BP 7021 Ouaga 03, Ouagadougou, Burkina Faso email: souaro@univ-ouaga.bf |
|---|---|
 |
Hamidou Toure Laboratoire d'Analyse Mathématique des Equations (LAME), UFR. Sciences Exactes et Appliquées, Université de Ouagadougou 03 BP 7021 Ouaga 03, Ouagadougou, Burkina Faso email: toureh@univ-ouaga.bf |
Return to the EJDE web page