In this note, we prove the existence of a solution to the semilinear second order ordinary differential equation
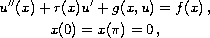
using a variational method and critical point theory.
Petr Tomiczek
Abstract:
In this note, we prove the existence of a solution to the
semilinear second order ordinary differential equation
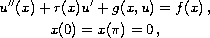
using a variational method and critical point theory.
Submitted April 24, 2007. Published May 29, 2007.
Math Subject Classifications: 34G20, 35A15, 34K10.
Key Words: Second order ODE; Dirichlet problem; variational method;
critical point.
Show me the PDF file (159K), TEX file, and other files for this article.
 |
Petr Tomiczek Department of Mathematics University of West Bohemia Univerzitni 22, 306 14 Plzen, Czech Republic email: tomiczek@kma.zcu.cz |
|---|
Return to the EJDE web page