Electron. J. Diff. Eqns.,
Vol. 2007(2007), No. 53, pp. 1-10.
A remark on ground state solutions for Lane-Emden-Fowler
equations with a convection term
Hongtao Xue, Zhijun Zhang
Abstract:
Via a sub-supersolution method and a perturbation argument, we
study the Lane-Emden-Fowler equation
![$$
-\Delta u =p(x)[g(u)+f(u)+|\nabla u|^q]
$$](gifs/aa.gif)
in
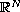 (
(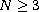 ),
where
),
where
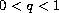 ,
,
 is a positive
weight such that
is a positive
weight such that
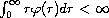 ,
where
,
where
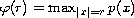 ,
,
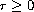 .
Under the hypotheses that both
.
Under the hypotheses that both
 and
and
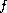 are sublinear, which include no
monotonicity on the functions
are sublinear, which include no
monotonicity on the functions
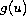 ,
,
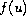 ,
,
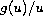 and
and
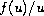 ,
we show the existence of ground state solutions.
,
we show the existence of ground state solutions.
Submitted January 6, 2007. Published April 10, 2007.
Math Subject Classifications: 35J60, 35B25, 35B50, 35R05.
Key Words: Ground state solution; Lane-Emden-Fowler equation;
convection term; maximum principle; existence;
sub-solution; super-solution.
Show me the
PDF file (242K),
TEX file, and other files for this article.
 |
Hongtao Xue
School of Mathematics and Informational Science
Yantai University, Yantai,
Shandong, 264005, China
email: ytxiaoxue@yahoo.com.cn
|
|---|
 |
Zhijun Zhang
Department of Mathematics and Informational Science
Yantai University, Yantai, Shandong, 264005, China
email: zhangzj@ytu.edu.cn |
|---|
Return to the EJDE web page
![$$
-\Delta u =p(x)[g(u)+f(u)+|\nabla u|^q]
$$](gifs/aa.gif)
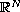 (
(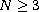 ),
where
),
where
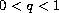 ,
,
 is a positive
weight such that
is a positive
weight such that
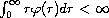 ,
where
,
where
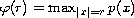 ,
,
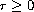 .
Under the hypotheses that both
.
Under the hypotheses that both
 and
and
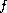 are sublinear, which include no
monotonicity on the functions
are sublinear, which include no
monotonicity on the functions
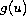 ,
,
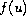 ,
,
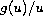 and
and
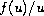 ,
we show the existence of ground state solutions.
,
we show the existence of ground state solutions.

