Electron. J. Diff. Eqns.,
Vol. 2006(2006), No. 39, pp. 1-10.
Positive solutions of singular fourth-order boundary-value problems
Yujun Cui, Yumei Zou
Abstract:
In this paper, we present necessary and sufficient conditions
for the existence of positive
![$C^3[0,1]\cap C^4(0,1)$](gifs/aa.gif) solutions
for the singular boundary-value problem
solutions
for the singular boundary-value problem
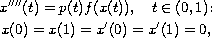
where
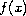 is either superlinear or sublinear,
is either superlinear or sublinear,
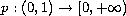 may be singular at both ends
may be singular at both ends
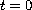 and
and
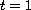 .
For this goal, we use fixed-point index results.
.
For this goal, we use fixed-point index results.
Submitted September 6, 2005. Published March 21, 2006
Math Subject Classifications: 34A34, 34B15, 45G15.
Key Words: Singular boundary value problem; fixed point theorem;
positive solution.
Show me the
PDF file (213K),
TEX file, and other files for this article.
 |
Yujun Cui
Department of Applied Mathematics
Shandong University of Science and technology
Qingdao, 266510, China
email: cyj720201@163.com |
|---|
| |
Yumei Zou
Department of Applied Mathematics
Shandong University of Science and technology
Qingdao, 266510, China
|
|---|
Return to the EJDE web page
![$C^3[0,1]\cap C^4(0,1)$](gifs/aa.gif) solutions
for the singular boundary-value problem
solutions
for the singular boundary-value problem
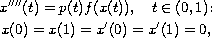
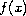 is either superlinear or sublinear,
is either superlinear or sublinear,
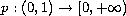 may be singular at both ends
may be singular at both ends
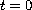 and
and
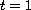 .
For this goal, we use fixed-point index results.
.
For this goal, we use fixed-point index results.
