 -Laplace equation with nonlinear
boundary conditions
-Laplace equation with nonlinear
boundary conditions
 -Laplace equation with nonlinear
boundary conditions
-Laplace equation with nonlinear
boundary conditions
Julian Fernandez Bonder
Abstract:
In this note, we show the existence of at least three nontrivial
solutions to the quasilinear elliptic equation
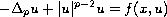
in a smooth bounded domain
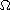 of
of
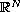 with nonlinear boundary conditions
with nonlinear boundary conditions
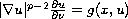 on
on
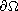 .
The proof is based on variational arguments.
.
The proof is based on variational arguments.
Submitted January 10, 2006. Published March 21, 2006.
Math Subject Classifications: 35J65, 35J20.
Key Words: p-Laplace equations; nonlinear boundary conditions;
variational methods.
Show me the PDF file (209K), TEX file, and other files for this article.
 |
Julián Fernández Bonder Departamento de Matemática, FCEyN UBA (1428) Buenos Aires, Argentina email: jfbonder@dm.uba.ar http://mate.dm.uba.ar/~jfbonder |
|---|
Return to the EJDE web page