Electron. J. Diff. Eqns.,
Vol. 2006(2006), No. 156, pp. 1-7.
Elasto-plastic torsion problem as an infinity Laplace's equation
Ahmed Addou, Abdeluaab Lidouh, Belkassem Seddoug
Abstract:
In this paper, we study a perturbed infinity Laplace's equation,
the perturbation corresponds to an Leray-Lions operator with
no coercivity assumption. We consider the case where data are
distributions or
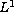 elements.
We show that this problem has an unique solution which is the solution to
the variational inequality arising in the elasto-plastic torsion problem,
associated with an operator
elements.
We show that this problem has an unique solution which is the solution to
the variational inequality arising in the elasto-plastic torsion problem,
associated with an operator
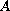 .
.
Submitted October 11, 2006. Published December 18, 2006.
Math Subject Classifications: 35J70, 35J85, 74C05.
Key Words: Infinity Laplace equation; elasto-plastic torsion problem;
variational inequality.
Show me the
PDF file (203K),
TEX file, and other files for this article.
 |
Ahmed Addou
Université Mohammed premier
Faculté des sciences
Oujda, Maroc
email: addou@sciences.univ-oujda.ac.ma
|
|---|
 |
Abdeluaab Lidouh
Université Mohammed premier
Faculté des sciences
Oujda, Maroc
email: lidouh@sciences.univ-oujda.ac.ma
|
|---|
 |
Belkassem Seddoug
Université Mohammed premier
Faculté des sciences
Oujda, Maroc
email: seddougbelkassem@yahoo.fr
|
|---|
Return to the EJDE web page
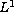 elements.
We show that this problem has an unique solution which is the solution to
the variational inequality arising in the elasto-plastic torsion problem,
associated with an operator
elements.
We show that this problem has an unique solution which is the solution to
the variational inequality arising in the elasto-plastic torsion problem,
associated with an operator
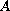 .
.


