Electron. J. Diff. Eqns., Vol. 2005(2005), No. 59, pp. 1-12.

Unique continuation property for the Kadomtsev-Petviashvili (KP-II) equation

Mahendra Panthee

Abstract:
We generalize a method introduced by Bourgain in \cite{Borg} based on complex analysis to address two spatial dimensional models and prove that if a sufficiently smooth solution to the initial value problem associated with the Kadomtsev-Petviashvili (KP-II) equation
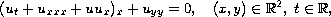
$$ (u_t+u_{xxx}+uu_{x})_{x} +u_{yy}=0, \quad (x, y) \in \mathbb{R}^2, \;t\in\mathbb{R}, $$
is supported compactly in a nontrivial time interval then it vanishes identically.

Submitted April 9, 2005. Published June 10, 2005.
Math Subject Classifications: 35Q35, 35Q53
Key Words: Dispersive equations; KP equation; unique continuation property; smooth solution; compact support.

Show me the PDF file (267K), TEX file, and other files for this article.

Mahendra Panthee
Central Department of Mathematics
Tribhuvan University, Kirtipur, Kathmandu, Nepal
and Department of Mathematics
CMAGDS, IST, 1049-001
Av. Rovisco Pais, Lisbon, Portugal
email: mpanthee@math.ist.utl.pt

Return to the EJDE web page