Electron. J. Diff. Eqns., Vol. 2005(2005), No. 04, pp. 1-11.
Positive solutions for elliptic equations with singular nonlinearity
Junping Shi, Miaoxin Yao
Abstract:
We study an elliptic boundary-value problem with singular
nonlinearity via the method of monotone iteration scheme:
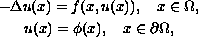
where
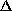 is the Laplacian operator,
is the Laplacian operator,
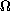 is a bounded domain
in
is a bounded domain
in
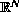 ,
,
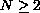 ,
,
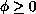 may take the value 0
on
may take the value 0
on
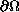 ,
and
,
and
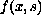 is possibly singular near
is possibly singular near
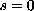 .
We prove the existence and the uniqueness of positive solutions
under a set of hypotheses that do not make neither monotonicity nor
strict positivity assumption on
.
We prove the existence and the uniqueness of positive solutions
under a set of hypotheses that do not make neither monotonicity nor
strict positivity assumption on
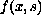 which improvements of
some previous results.
which improvements of
some previous results.
Submitted August 15, 2004. Published January 2, 2005.
Math Subject Classifications: 35J25, 35J60.
Key Words: Singular nonlineararity; elliptic equation; positive solution;
monotonic iteration.
Show me the
PDF file (247K),
TEX file, and other files for this article.
 |
Junping Shi
Department of Mathematics, College of William and Mary
Williamsburg, VA 23187, USA
Department of Mathematics, Harbin Normal University
Harbin, Heilongjiang, China
email: shij@math.wm.edu |
|---|
 |
Miaoxin Yao
Department of Mathematics, Tianjin University
and Liu Hui Center for Applied Mathematics
Nankai University & Tianjin University
Tianjin, 300072, China |
|---|
Return to the EJDE web page
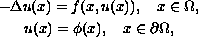
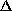 is the Laplacian operator,
is the Laplacian operator,
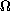 is a bounded domain
in
is a bounded domain
in
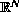 ,
,
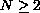 ,
,
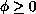 may take the value 0
on
may take the value 0
on
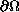 ,
and
,
and
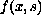 is possibly singular near
is possibly singular near
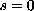 .
We prove the existence and the uniqueness of positive solutions
under a set of hypotheses that do not make neither monotonicity nor
strict positivity assumption on
.
We prove the existence and the uniqueness of positive solutions
under a set of hypotheses that do not make neither monotonicity nor
strict positivity assumption on
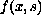 which improvements of
some previous results.
which improvements of
some previous results.

