Electron. J. Diff. Eqns., Vol. 2004(2004), No. 147, pp. 1-18.
Generalized scalar curvature type equation
on complete Riemannian manifolds
Mohammed Benalili, Youssef Maliki
Abstract:
In this work, we investigate positive solutions for a quasilinear
elliptic equation on complete manifold
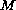 .
This equation extends to the
.
This equation extends to the
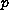 -Laplacian
the equation of the prescribed scalar curvature.
A minimizing sequence is constructed which converges to a non trivial
solution belonging to
-Laplacian
the equation of the prescribed scalar curvature.
A minimizing sequence is constructed which converges to a non trivial
solution belonging to
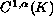 for any compact set
for any compact set
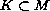 and some
and some
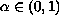 .
.
Submitted November 26, 2004. Published December 13, 2004.
Math Subject Classifications: 58J05.
Key Words: Complete manifolds; p-Laplacian; critical Sobolev exponent.
Show me the
PDF file (264K),
TEX file, and other files for this article.
 |
Mohamed Benalili
Faculté des Sciences, Dept. de Mathématiques
B. P. 119, Université Aboubekr Belkaïd, Tlemcen,
Algerie
email: m_benalili@mail.univ-tlemcen.dz |
|---|
 |
Youssef Maliki
Faculté des Sciences, Dept. de Mathématiques
B. P. 119, Université Aboubekr Belkaïd, Tlemcen,
Algerie
email: m-youssef@mail.univ-tlemcen.dz |
|---|
Return to the EJDE web page
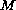 .
This equation extends to the
.
This equation extends to the
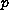 -Laplacian
the equation of the prescribed scalar curvature.
A minimizing sequence is constructed which converges to a non trivial
solution belonging to
-Laplacian
the equation of the prescribed scalar curvature.
A minimizing sequence is constructed which converges to a non trivial
solution belonging to
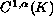 for any compact set
for any compact set
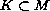 and some
and some
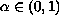 .
.

