Electron. J. Diff. Eqns., Vol. 2004(2004), No. 145, pp. 1-10.
Estimates for the mixed derivatives of the Green functions
on homogeneous manifolds of negative curvature
Roman Urban
Abstract:
We consider the Green functions for second-order left-invariant
differential operators on homogeneous manifolds of negative
curvature, being a semi-direct product of a nilpotent Lie group
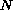 and
and
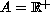 . We obtain estimates for mixed derivatives
of the Green functions both in the coercive and non-coercive case.
The current paper completes the previous results obtained by the
author in a series of papers [14,15,16,19].
. We obtain estimates for mixed derivatives
of the Green functions both in the coercive and non-coercive case.
The current paper completes the previous results obtained by the
author in a series of papers [14,15,16,19].
Submitted October 17, 2003. Published December 7, 2004.
Math Subject Classifications: 22E25, 43A85, 53C30.
Key Words: Green function; second-order differential operators;
NA groups; Bessel process; evolutions on nilpotent Lie groups.
Show me the
PDF file (244K),
TEX file, and other files for this article.
 |
Roman Urban
Institute of Mathematics
University of Wroclaw
Pl. Grunwaldzki 2/4
50-384 Wroclaw, Poland
email: urban@math.uni.wroc.pl |
|---|
Return to the EJDE web page
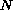 and
and
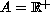 . We obtain estimates for mixed derivatives
of the Green functions both in the coercive and non-coercive case.
The current paper completes the previous results obtained by the
author in a series of papers [14,15,16,19].
. We obtain estimates for mixed derivatives
of the Green functions both in the coercive and non-coercive case.
The current paper completes the previous results obtained by the
author in a series of papers [14,15,16,19].
