A new fixed point theorem on double cones is applied to obtain the existence of at least two positive solutions to
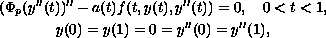
where
![$f:[0,1]\times[0,\infty)\times(-\infty,0]\to R,
a\in L^1([0,1],(0,\infty)) $](gifs/ab.gif) . We also give some examples to
illustrate our results.
. We also give some examples to
illustrate our results.
Yu Tian, Weigao Ge
Abstract:
A new fixed point theorem on double cones is applied to obtain
the existence of at least two positive solutions to
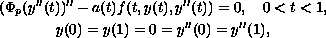
where
![$f:[0,1]\times[0,\infty)\times(-\infty,0]\to R,
a\in L^1([0,1],(0,\infty)) $](gifs/ab.gif) . We also give some examples to
illustrate our results.
. We also give some examples to
illustrate our results.
Submitted August 28, 2004. Published December 3, 2004.
Math Subject Classifications: 34B10, 34B15.
Key Words: Fourth-order two-point boundary-value problem; fixed
point theorem; double cones; positive solution.
Show me the PDF file (199K), TEX file, and other files for this article.
 |
Yu Tian Department of Applied Mathematics Beijing Institute of Technology, Beijing 100081, China email: tianyu2992@163.com |
|---|---|
| Weigao Ge Department of Applied Mathematics Beijing Institute of Technology, Beijing 100081, China email: gew@bit.edu.cn |
Return to the EJDE web page