By means of variational structure and
 group
index theory, we obtain multiple solutions for the second-order
differential equation
group
index theory, we obtain multiple solutions for the second-order
differential equation
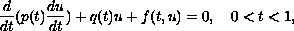
subject to one of the following two sets of boundary conditions:
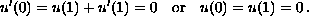
Xiao-Bao Shu, Yuan-Tong Xu
Abstract:
By means of variational structure and
 group
index theory, we obtain multiple solutions for the second-order
differential equation
group
index theory, we obtain multiple solutions for the second-order
differential equation
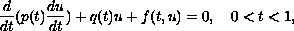
subject to one of the following two sets of boundary conditions:
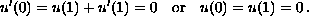
Submitted October 5, 2004. Published November 25, 2004.
Math Subject Classifications: 34B15, 34B05, 65K10, 34B24.
Key Words: Variational structure;
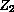 group index theory;
critical points; boundary value problems.
group index theory;
critical points; boundary value problems.
Show me the PDF file (249K), TEX file, and other files for this article.
 |
Xiao-Bao Shu Department of Mathematics Sun Yat-Sen University Guangzhou, 510275, China email: sxb0221@163.com |
|---|---|
 |
Yuan-Tong Xu Department of Mathematics Sun Yat-Sen University Guangzhou, 510275, China email: xyt@zsu.edu.cn |
Return to the EJDE web page