Electron. J. Diff. Eqns., Vol. 2004(2004), No. 121, pp. 1-22.
Concentration phenomena for fourth-order elliptic
equations with critical exponent
Mokhless Hammami
Abstract:
We consider the nonlinear equation
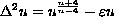
with
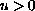 in
in
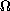 and
and
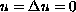 on
on
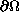 .
Where
.
Where
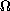 is a smooth bounded domain in
is a smooth bounded domain in
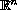 ,
,
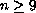 ,
and
,
and
 is a small positive parameter.
We study the existence of solutions which
concentrate around one or two points of
is a small positive parameter.
We study the existence of solutions which
concentrate around one or two points of
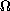 .
We show that this problem has no solutions that concentrate
around a point of
.
We show that this problem has no solutions that concentrate
around a point of
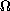 as
as
 approaches 0.
In contrast to this, we construct a domain for which
there exists a family of solutions which blow-up and concentrate
in two different points of
approaches 0.
In contrast to this, we construct a domain for which
there exists a family of solutions which blow-up and concentrate
in two different points of
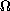 as
as
 approaches 0.
approaches 0.
Submitted August 25, 2004. Published October 14, 2004.
Math Subject Classifications: 35J65, 35J40, 58E05.
Key Words: Fourth order elliptic equations; critical Sobolev exponent;
blowup solution.
Show me the
PDF file (345K),
TEX file, and other files for this article.
 |
Mokhless Hammami
Département de Mathématiques
Faculté des Sciences de Sfax
Route Soukra, 3018, Sfax, Tunisia
email: Mokhless.Hammami@fss.rnu.tn |
|---|
Return to the EJDE web page
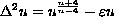
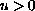 in
in
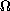 and
and
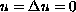 on
on
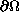 .
Where
.
Where
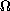 is a smooth bounded domain in
is a smooth bounded domain in
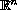 ,
,
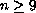 ,
and
,
and
 is a small positive parameter.
We study the existence of solutions which
concentrate around one or two points of
is a small positive parameter.
We study the existence of solutions which
concentrate around one or two points of
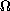 .
We show that this problem has no solutions that concentrate
around a point of
.
We show that this problem has no solutions that concentrate
around a point of
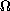 as
as
 approaches 0.
In contrast to this, we construct a domain for which
there exists a family of solutions which blow-up and concentrate
in two different points of
approaches 0.
In contrast to this, we construct a domain for which
there exists a family of solutions which blow-up and concentrate
in two different points of
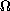 as
as
 approaches 0.
approaches 0.
