Electron. J. Diff. Eqns.,
Vol. 2003(2003), No. 84, pp. 1-8.
Asymptotic behavior for a heat conduction
problem with perfect-contact boundary condition
Andres Barrea & Cristina Turner
Abstract:
In this paper we consider the heat conduction problem for
a slab represented by the interval
![$[0,1]$](gifs/aa.gif) .
The initial temperature is a positive constant, the flux
at the left end is also a positive constant, and at
the right end there is a perfect contact condition:
.
The initial temperature is a positive constant, the flux
at the left end is also a positive constant, and at
the right end there is a perfect contact condition:
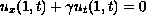 .
We analyze the asymptotic behavior of these problems as
.
We analyze the asymptotic behavior of these problems as
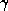 approaches infinity, and present some numerical
calculations.
approaches infinity, and present some numerical
calculations.
Submitted July 15, 2002. Published August 14, 2003.
Math Subject Classifications: 35K60, 35R35.
Key Words: Heat conduction, phase change, free boundary, perfect contact.
Show me the
PDF file (307K),
TEX file, and other files for this article.
 |
Andres Barrea
Fa.M.A.F.- Universidad Nacional de Cordoba
CIEM-CONICET, Cordoba, Argentina
email: abarrea@mate.uncor.edu
|
|---|
 |
Cristina Turner
Fa.M.A.F.- Universidad Nacional de Cordoba
CIEM-CONICET, Cordoba, Argentina
email: turner@mate.uncor.edu
|
|---|
Return to the EJDE web page
![$[0,1]$](gifs/aa.gif) .
The initial temperature is a positive constant, the flux
at the left end is also a positive constant, and at
the right end there is a perfect contact condition:
.
The initial temperature is a positive constant, the flux
at the left end is also a positive constant, and at
the right end there is a perfect contact condition:
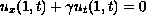 .
We analyze the asymptotic behavior of these problems as
.
We analyze the asymptotic behavior of these problems as
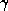 approaches infinity, and present some numerical
calculations.
approaches infinity, and present some numerical
calculations.

