Electron. J. Diff. Eqns.,
Vol. 2003(2003), No. 34, pp. 1-7.
Centering conditions for planar septic systems
Evgenii P. Volokitin
Abstract:
We find centering conditions for the following
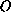 -symmetric system
of degree 7:
-symmetric system
of degree 7:
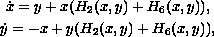
where
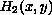 and
and
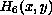 are homogeneous polynomials
of degrees 2 and 6, respectively.
In some cases, we can find commuting systems and first integrals for the
original system. We also study the geometry of the central region.
are homogeneous polynomials
of degrees 2 and 6, respectively.
In some cases, we can find commuting systems and first integrals for the
original system. We also study the geometry of the central region.
Submitted August 22, 2002. Published April 3, 2003.
Math Subject Classifications: 34C05, 34C25.
Key Words: centering conditions, isochronicity, commutativity.
Show me the
PDF file (162K),
TEX file, and other files for this article.
 |
Evgenii P. Volokitin
Sobolev Institute of Mathematics
Novosibirsk, 630090, Russia
email: volok@math.nsc.ru |
|---|
Return to the EJDE web page
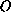 -symmetric system
of degree 7:
-symmetric system
of degree 7:
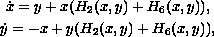
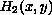 and
and
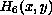 are homogeneous polynomials
of degrees 2 and 6, respectively.
In some cases, we can find commuting systems and first integrals for the
original system. We also study the geometry of the central region.
are homogeneous polynomials
of degrees 2 and 6, respectively.
In some cases, we can find commuting systems and first integrals for the
original system. We also study the geometry of the central region.
