Electron. J. Diff. Eqns.,
Vol. 2003(2003), No. 125, pp. 1-7.
Exponential stability of linear and almost periodic
systems on Banach spaces
Constantin Buse & Vasile Lupulescu
Abstract:
Let
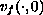 the mild solution of the well-posed
inhomogeneous Cauchy problem
the mild solution of the well-posed
inhomogeneous Cauchy problem
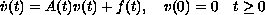
on a complex Banach space
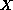 , where
, where
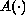 is an almost
periodic (possible unbounded) operator-valued function.
We prove that
is an almost
periodic (possible unbounded) operator-valued function.
We prove that
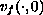 )
belongs to a suitable subspace
of bounded and uniformly continuous functions if and only if
for each
)
belongs to a suitable subspace
of bounded and uniformly continuous functions if and only if
for each
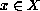 the solution of the homogeneous Cauchy problem
the solution of the homogeneous Cauchy problem

is uniformly exponentially stable. Our approach is based on the
spectral theory of evolution semigroups.
Submitted November 13, 2003. Published December 16, 2003.
Math Subject Classifications: 35B10, 35B15, 35B40, 47A10, 47D03.
Key Words: Almost periodic functions, uniform exponential stability,
evolution semigroups.
Show me the
PDF file (207K),
TEX file, and other files for this article.
 |
Constantin Buse
Department of Mathematics
West University of Timisoara
Bd. V. Parvan 4, Timisoara, Romania
e-mail: buse@hilbert.math.uvt.ro
|
|---|
 |
Vasile Lupulescu
Department of Mathematics
"Constantin Brancusi"- University of Tg. Jiu
Bd. Republicii, No. 1, Tg. Jiu, Romania
email: vasile@utgjiu.ro
|
|---|
Return to the EJDE web page
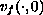 the mild solution of the well-posed
inhomogeneous Cauchy problem
the mild solution of the well-posed
inhomogeneous Cauchy problem
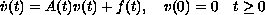
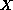 , where
, where
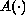 is an almost
periodic (possible unbounded) operator-valued function.
We prove that
is an almost
periodic (possible unbounded) operator-valued function.
We prove that
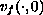 )
belongs to a suitable subspace
of bounded and uniformly continuous functions if and only if
for each
)
belongs to a suitable subspace
of bounded and uniformly continuous functions if and only if
for each
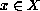 the solution of the homogeneous Cauchy problem
the solution of the homogeneous Cauchy problem


