Electron. J. Diff. Eqns., Vol. 2002(2002), No. 88, pp. 1-13.
Existence of global solutions to reaction-diffusion systems with
nonhomogeneous boundary conditions via a Lyapunov functional
Said Kouachi
Abstract:
Most publications on reaction-diffusion systems of
 components
(
components
(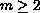 )
impose
)
impose
 inequalities to the reaction terms,
to prove existence of global solutions
(see Martin and Pierre [10] and Hollis [4]).
The purpose of this paper is to prove existence of a global solution
using only one inequality in the case of 3 component systems.
Our technique is based on the construction of polynomial functionals
(according to solutions of the reaction-diffusion equations)
which give, using the well known regularizing effect,
the global existence. This result generalizes those obtained
recently by Kouachi [6] and independently by Malham
and Xin [9].
inequalities to the reaction terms,
to prove existence of global solutions
(see Martin and Pierre [10] and Hollis [4]).
The purpose of this paper is to prove existence of a global solution
using only one inequality in the case of 3 component systems.
Our technique is based on the construction of polynomial functionals
(according to solutions of the reaction-diffusion equations)
which give, using the well known regularizing effect,
the global existence. This result generalizes those obtained
recently by Kouachi [6] and independently by Malham
and Xin [9].
Submitted December 13, 2001. Published October 16, 2002.
Math Subject Classifications: 35K45, 35K57.
Key Words: Reaction diffusion systems, Lyapunov functionals,
global existence
Show me the
PDF file (243K),
TEX file, and other files for this article.
 |
Said Kouachi
Centre Univ. Tebessa, Dept. Mathematiques,
12002, Tebessa, Algerie
and
Lab. Mathemathiques, Universite d'Annaba,
B. P. 12, Annaba, 23200, Algerie
e-mail: kouachi.said@caramail.com
|
|---|
Return to the EJDE web page
 components
(
components
(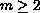 )
impose
)
impose
 inequalities to the reaction terms,
to prove existence of global solutions
(see Martin and Pierre [10] and Hollis [4]).
The purpose of this paper is to prove existence of a global solution
using only one inequality in the case of 3 component systems.
Our technique is based on the construction of polynomial functionals
(according to solutions of the reaction-diffusion equations)
which give, using the well known regularizing effect,
the global existence. This result generalizes those obtained
recently by Kouachi [6] and independently by Malham
and Xin [9].
inequalities to the reaction terms,
to prove existence of global solutions
(see Martin and Pierre [10] and Hollis [4]).
The purpose of this paper is to prove existence of a global solution
using only one inequality in the case of 3 component systems.
Our technique is based on the construction of polynomial functionals
(according to solutions of the reaction-diffusion equations)
which give, using the well known regularizing effect,
the global existence. This result generalizes those obtained
recently by Kouachi [6] and independently by Malham
and Xin [9].
