We study the Dirichlet Problem associated to the quasilinear elliptic problem
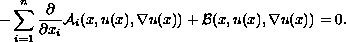
Then we define a potential theory related to this problem and we show that the sheaf of continuous solutions satisfies the Bauer axiomatic theory.
Azeddine Baalal & Nedra BelHaj Rhouma
Abstract:
We study the Dirichlet Problem associated to the quasilinear
elliptic problem
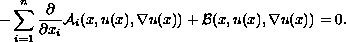
Then we define a potential theory related to this problem and we
show that the sheaf of continuous solutions satisfies the Bauer
axiomatic theory.
Submitted April 9, 2002. Published October 2, 2002.
Math Subject Classifications: 31C15, 35B65, 35J60.
Key Words: Supersolution, Dirichlet problem, obstacle problem,
nonlinear potential theory.
Show me the PDF file (309K), TEX file, and other files for this article.
| Azeddine Baalal Departement de Mathematiques et d'Informatique, Faculte des Sciences Ain Chock, Km 8 Route El Jadida BP 5366 Maarif, Casablanca, Maroc. E-mail: baalal@facsc-achok.ac.ma | |
 |
Nedra BelHaj Rhouma Institut Preparatoire aux Etudes d'Ingenieurs de Tunis, 2 Rue Jawaher Lel Nehru, 1008 Montfleury, Tunis, Tunisie. E-mail: Nedra.BelHajRhouma@ipeit.rnu.tn |
Return to the EJDE web page