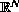Electron. J. Diff. Eqns., Vol. 2002(2002), No. 25, pp. 1-15.

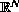Existence and multiplicity results for nonlinear elliptic problems in $\mathbb{R}^N$ with an indefinite functional

David G. Costa, Yuxia Guo, & Miguel Ramos

Abstract:
We prove the existence of a nontrivial solution for the nonlinear elliptic problem
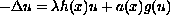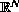
$-\Delta u=\lambda h(x)u + a(x)g(u)$ in $\mathbb{R}^N$
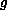where $g$ is superlinear near zero and near infinity, 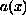$a(x)$ changes sign, 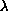$\lambda $ is positive, and 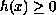$h(x)\geq 0$ is a weight function. For 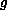$g$ odd, we prove the existence of an infinite number of solutions.

Submitted June 23, 2001. Published March 4, 2002.
Math Subject Classifications: 35J25, 35J20, 58E05.
Key Words: Superlinear elliptic problems, Morse index, minimax methods.

Show me the PDF file (298K), TEX file, and other files for this article.

David G. Costa
Dept. of Math. Sciences, University of Nevada,
Las Vegas, NV 89154-4020, USA
e-mail: costa@unlv.edu

Yuxia Guo
Chinese Academy of Sciences
Beijing 100080, China
e-mail: yxguo@lsc02.iss.ac.cn

Miguel Ramos
CMAF, Univ. de Lisboa
Av. Prof. Gama Pinto, 1649-003 Lisboa, Portugal
e-mail: mramos@lmc.fc.ul.pt

Return to the EJDE web page