Electron. J. Diff. Eqns., Vol. 2002(2002), No. 14, pp. 1-14.
A class of nonlinear elliptic variational inequalities:
qualitative properties and existence of solutions
Luka Korkut, Mervan Pasic, & Darko Zubrinic
Abstract:
We study a class of nonlinear elliptic variational inequalities
in divergence form. In the recent paper [6], we obtained
results on the local control of essential infimum and supremum of
solutions of quasilinear elliptic equations, and here we extend this
point of view to the case of variational inequalities.
It implies a new qualitative property of solutions in
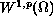 which we call ``jumping over the control obstacle.''
Using the Schwarz symmetrization technique, we give an existence
and symmetrization theorems in
which we call ``jumping over the control obstacle.''
Using the Schwarz symmetrization technique, we give an existence
and symmetrization theorems in
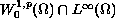 which agree completely with previous qualitative results. Also we consider
generating singularities of weak solutions in
which agree completely with previous qualitative results. Also we consider
generating singularities of weak solutions in
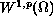 of variational inequalities.
of variational inequalities.
Submitted December 15, 2001. Published February 9, 2002.
Math Subject Classifications: 35J65, 35J85, 35B05.
Key Words: variational inequalities, double obstacle,
qualitative properties, Schwarz symmetrization,
generating singularities.
Show me the
PDF file (270K),
TEX file, and other files for this article.
| |
Luka Korkut
Department of mathematics
Faculty of Electrical Engineering and Computing
University of Zagreb
Unska 3, 10000 Zagreb, Croatia
e-mail: luka.korkut@fer.hr |
|---|
 |
Mervan Pasic
Department of mathematics
Faculty of Electrical Engineering and Computing
University of Zagreb
Unska 3, 10000 Zagreb, Croatia
e-mail: mervan.pasic@fer.hr |
|---|
| |
Darko Zubrinic
Department of mathematics
Faculty of Electrical Engineering and Computing
University of Zagreb
Unska 3, 10000 Zagreb, Croatia
e-mail: darko.zubrinic@fer.hr |
|---|
Return to the EJDE web page
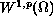 which we call ``jumping over the control obstacle.''
Using the Schwarz symmetrization technique, we give an existence
and symmetrization theorems in
which we call ``jumping over the control obstacle.''
Using the Schwarz symmetrization technique, we give an existence
and symmetrization theorems in
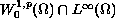 which agree completely with previous qualitative results. Also we consider
generating singularities of weak solutions in
which agree completely with previous qualitative results. Also we consider
generating singularities of weak solutions in
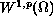 of variational inequalities.
of variational inequalities.
