Electron. J. Diff. Eqns., Vol. 2002(2002), No. 13, pp. 1-29.
Some remarks on the Melnikov function
Flaviano Battelli & Michal Feckan
Abstract:
We study the Melnikov function associated with a periodic
perturbation of a differential equation having a homoclinic orbit.
Our main interest is the characterization of perturbations that
give rise to vanishing or non-vanishing of the Melnikov function.
For this purpose we show that, in some cases, the Fourier coefficients
of the Melkinov function can be evaluated by means of the calculus of
residues. We apply this result, among other things, to the construction
of a second-order equation whose Melnikov function vanishes identically
for any
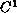 ,
,
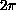 -periodic
perturbation. Then we study the second
order Melnikov function of the perturbed equation, and prove it is
non-vanishing for a large class of perturbations.
-periodic
perturbation. Then we study the second
order Melnikov function of the perturbed equation, and prove it is
non-vanishing for a large class of perturbations.
Submitted November 27, 2001. Published February 7, 2002.
Math Subject Classifications: 34C23, 34C37.
Key Words: Melnikov function, residues, Fourier coefficients.
Show me the
PDF file (330K),
TEX file, and other files for this article.
 |
Flaviano Battelli
Dipartimento di Matematica ``V. Volterra'',
Facolta di Ingegneria - Universita di Ancona,
Via Brecce Bianche 1, 60131 Ancona - Italy
e-mail: fbat@dipmat.unian.it |
|---|
 |
Michal Feckan
Department of Mathematical Analysis, Comenius University,
Mlynska dolina, 842 48 Bratislava - Slovakia
e-mail: Michal.Feckan@fmph.uniba.sk |
|---|
Return to the EJDE web page
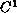 ,
,
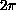 -periodic
perturbation. Then we study the second
order Melnikov function of the perturbed equation, and prove it is
non-vanishing for a large class of perturbations.
-periodic
perturbation. Then we study the second
order Melnikov function of the perturbed equation, and prove it is
non-vanishing for a large class of perturbations.

