Electron. J. Diff. Eqns., Vol. 2001(2001), No. 46, pp. 1-20.
A unique continuation property for linear elliptic systems and
nonresonance problems
A. Anane, O. Chakrone, Z. El Allali, & I. Hadi
Abstract:
The aim of this paper is to study the existence of solutions for a
quasilinear elliptic system where the nonlinear term is a Caratheodory
function on a bounded domain of
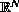 ,
by proving the well
known unique continuation property for elliptic system in all dimensions:
1, 2, 3, ... and the strict monotonocity of eigensurfaces.
These properties let us to consider the above problem as a nonresonance
problem.
,
by proving the well
known unique continuation property for elliptic system in all dimensions:
1, 2, 3, ... and the strict monotonocity of eigensurfaces.
These properties let us to consider the above problem as a nonresonance
problem.
Submitted January 28, 2000. Published June 20, 2001.
Math Subject Classifications: 35J05, 35J45, 35J65.
Key Words: Unique continuation, eigensurfaces, nonresonance problem.
Show me the
PDF file (304K),
TEX file, and other files for this article.
 |
A. Anane
Departement de Mathematiques et Informatique
Faculte des Sciences
Universite Mohamed 1, Oujda, Maroc
e-mail: anane@sciences.univ-oujda.ac.ma |
 |
O. Chakrone
Departement de Mathematiques et Informatique
Faculte des Sciences
Universite Mohamed 1, Oujda, Maroc
chakrone@sciences.univ-oujda.ac.ma |
 |
Z. El Allali
Departement de Mathematiques et Informatique
Faculte des Sciences
Universite Mohamed 1, Oujda, Maroc
zakaria@sciences.univ-oujda.ac.ma |
 |
Islam Eddine Hadi
Departement de Mathematiques et Informatique
Faculte des Sciences
Universite Mohamed 1, Oujda, Maroc
hadi@sciences.univ-oujda.ac.ma |
Return to the EJDE web page
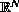 ,
by proving the well
known unique continuation property for elliptic system in all dimensions:
1, 2, 3, ... and the strict monotonocity of eigensurfaces.
These properties let us to consider the above problem as a nonresonance
problem.
,
by proving the well
known unique continuation property for elliptic system in all dimensions:
1, 2, 3, ... and the strict monotonocity of eigensurfaces.
These properties let us to consider the above problem as a nonresonance
problem.



