We prove the existence of regular solutions for the quasi-linear evolution

where
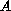 is the infinitesimal generator of an analytic semigroup
of bounded linear operators defined on a Banach space and the functions
is the infinitesimal generator of an analytic semigroup
of bounded linear operators defined on a Banach space and the functions
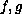 are continuous.
are continuous.
Eduardo Hernandez M.
Abstract:
We prove the existence of regular solutions for the
quasi-linear evolution

where
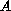 is the infinitesimal generator of an analytic semigroup
of bounded linear operators defined on a Banach space and the functions
is the infinitesimal generator of an analytic semigroup
of bounded linear operators defined on a Banach space and the functions
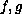 are continuous.
are continuous.
Submitted December 14, 2000. Published April 10, 2001.
Math Subject Classifications: 35A05, 34G20, 34A09.
Key Words: Banach spaces, semigroup of linear operators,
abstract differential equations,
fractional powers of closed operators, regular solutions.
Show me the PDF file (231K), TEX file, and other files for this article.
 |
Eduardo Hernandez M. Departamento de Matematica Instituto de Ciencias Matematicas de Sao Carlos Universidade de Sao Paulo Caixa Postal 668 13560-970 Sao Carlos, SP. Brazil e-mail: lalohm@icmc.sc.usp.br |
|---|
Return to the EJDE web page