Electron. J. Diff. Eqns., Vol. 2000(2000), No. 61, pp. 1-15
Quantitative uniqueness and vortex degree estimates
for solutions of the Ginzburg-Landau equation
Igor Kukavica
Abstract:
In this paper, we provide a sharp upper bound for the
maximal order of vanishing for non-minimizing solutions
of the Ginzburg-Landau equation
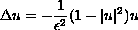
which improves our previous result [12]. An
application of this result is a sharp upper bound for the
degree of any vortex.
We treat Dirichlet (homogeneous and non-homogeneous)
as well as Neumann boundary conditions.
Submitted June 23, 2000. Published October 2, 2000.
Math Subject Classifications: 35B05, 35J25, 35J60, 35J65, 35Q35.
Key Words: Unique continuation, vortices, Ginzburg-Landau equation.
Show me the
PDF file (163K),
TEX file, and other files for this article.
 |
Igor Kukavica
Department of Mathematics
University of Southern California
Los Angeles, CA 90089
e-mail: kukavica@math.usc.edu |
Return to the EJDE web page