Electron.n. J. Diff. Eqns., Vol. 1999(1999), No. 22, pp. 1-15.
Antimaximum principle for elliptic problems with weight
T. Godoy, J.-P. Gossez, & S. Paczka
Abstract:
This paper is concerned with the antimaximum principle for the linear
problem with weight
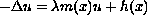
under Dirichlet or Neumann boundary conditions.
We investigate the following three questions:
Where exactly can this principle hold?
If it holds, does it hold uniformly or not?
If it holds uniformly, what is the exact interval of uniformity?
We will in particular obtain a variational characterization of
this interval of uniformity.
Submitted February 2, 1999. Published June 17, 1999.
Math Subject Classification: 35J20, 35P05.
Key Words: Antimaximum principle, indefinite weight, Fucik spectrum.
Show me the
PDF file (170K),
TEX file, and other files for this article.
T. Godoy
FAMAF, Univ. Nacional Cordoba
Ciudad universitaria, 5000 Cordoba, Argentina
e-mail: godoy@mate.uncor.edu
J.-P. Gossez
Dep. Mathematique, C.P. 214
Universite Libre de Bruxelles
1050 Bruxelles, Belgique
e-mail: gossez@ulb.ac.be
S. Paczka
FAMAF, Univ. Nacional Cordoba
Ciudad universitaria, 5000 Cordoba, Argentina
e-mail: paczka@mate.uncor.edu
Return to the EJDE web page