Electron.n. J. Diff. Eqns., Vol. 1999(1999), No. 21, pp. 1-27.
Multiplicity of solutions for quasilinear elliptic boundary-value problems
Idris Addou
Abstract:
This paper is concerned with the existence of multiple solutions to the
boundary-value problem
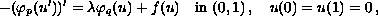
where
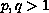 ,
,
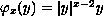 ,
,
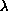 is a real parameter, and
is a real parameter, and
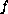 is a function which may be
sublinear, superlinear, or asymmetric.
We use the time map method for showing the existence of solutions.
is a function which may be
sublinear, superlinear, or asymmetric.
We use the time map method for showing the existence of solutions.
Submitted January 21, 1999. Published June 16, 1999.
Math Subject Classification: 34B15.
Key Words: Multiple solutions, elliptic boundary-value problems.
Show me the
PDF file (229K),
TEX file, and other files for this article.
 |
Idris Addou
USTHB, Institut de Mathematiques
El-Alia, B.P. no. 32 Bab-Ezzouar
16111, Alger, Algerie.
e-mail: idrisaddou@hotmail.com |
Return to the EJDE web page
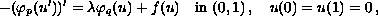
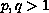 ,
,
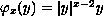 ,
,
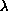 is a real parameter, and
is a real parameter, and
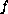 is a function which may be
sublinear, superlinear, or asymmetric.
We use the time map method for showing the existence of solutions.
is a function which may be
sublinear, superlinear, or asymmetric.
We use the time map method for showing the existence of solutions. 