Electron. J. Diff. Eqns., Vol. 1998(1998), No. 15, pp. 1-23.
Stability estimate for strong solutions of the Navier-Stokes system
and its applications
Tadashi Kawanago
Abstract:
We obtain a `stability estimate' for strong solutions
of the Navier-Stokes system, which is an
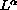 -version,
-version,
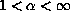 ,
of the estimate that Serrin [Se] used in obtaining
uniqueness of weak solutions to the Navier-Stokes system.
By applying this estimate, we obtain new results in stability and
uniqueness of solutions, and non-blowup conditions for strong solutions.
,
of the estimate that Serrin [Se] used in obtaining
uniqueness of weak solutions to the Navier-Stokes system.
By applying this estimate, we obtain new results in stability and
uniqueness of solutions, and non-blowup conditions for strong solutions.
Submitted February 17, 1998. Published June 3, 1998.
Math Subject Classification: 35Q30, 76D05.
Key Words: Navier-Stokes system, strong solutions, stability, uniqueness,
non-blowup condition.
Show me the
PDF file (214 KB),
TEX file, and other files for this article.
Tadashi Kawanago
Department of Applied Mathematics
Faculty of Engineering, Shizuoka University
Hamamatsu 432, Japan
E-mail tstkawa@eng.shizuoka.ac.jp
Return to the EJDE home page
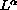 -version,
-version,
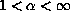 ,
of the estimate that Serrin [Se] used in obtaining
uniqueness of weak solutions to the Navier-Stokes system.
By applying this estimate, we obtain new results in stability and
uniqueness of solutions, and non-blowup conditions for strong solutions.
,
of the estimate that Serrin [Se] used in obtaining
uniqueness of weak solutions to the Navier-Stokes system.
By applying this estimate, we obtain new results in stability and
uniqueness of solutions, and non-blowup conditions for strong solutions.