We study nonexistence and removability results for nonnegative sub-solutions to

where
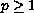 ,
,
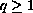 ,
,
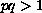 , and
, and
Cecilia S. Yarur
Abstract:
We study nonexistence and removability results for nonnegative
sub-solutions to

where
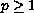 ,
,
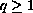 ,
,
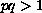 , and
, and
Submitted June 6, 1996. Published September 6, 1996.
Math Subject Class.: 35J20, 31A35.
Key Words: Elliptic systems, Removable singularity, Biharmonic equation.
Show me the PDF file (225 KB), TEX file, and other files for this article.