Electron. J. Diff. Eqns.,
Vol. 1995(1995), No. 09, pp. 1-15.
Singularity Formation in Systems of Non-strictly
Hyperbolic Equations
R. Saxton & V. Vinod
Abstract:
We analyze finite time singularity formation for two systems
of hyperbolic equations. Our results extend previous proofs of breakdown
concerning
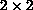 non-strictly hyperbolic systems to
non-strictly hyperbolic systems to
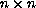 systems, and to a situation where, additionally, the condition of
genuine nonlinearity is violated throughout phase space. The systems
we consider include as special cases those examined by Keyfitz and
Kranzer and by Serre. They take the form
systems, and to a situation where, additionally, the condition of
genuine nonlinearity is violated throughout phase space. The systems
we consider include as special cases those examined by Keyfitz and
Kranzer and by Serre. They take the form
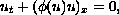
where
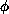 is a scalar-valued function of the n-dimensional
vector
is a scalar-valued function of the n-dimensional
vector
 , and
, and
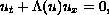
under the assumption
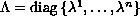 with
with
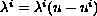 ,
where
,
where
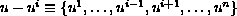 .
.
Submitted June 12, 1995. Published: June 28, 1995.
Math Subject Classification: 35L45, 35L65, 35L67, 35L80.
Key Words: Finite time breakdown, non-strict hyperbolicity,
linear degeneracy.
Show me the
PDF file (221 KB),
TEX file, and other files for this article.
R. Saxton (rsaxton@math.uno.edu)
V. Vinod (vvinod@math.uno.edu)
Department of Mathematics,
University of New Orleans,
New Orleans, LA 70148, USA
Return to the EJDE home page.
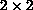 non-strictly hyperbolic systems to
non-strictly hyperbolic systems to
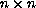 systems, and to a situation where, additionally, the condition of
genuine nonlinearity is violated throughout phase space. The systems
we consider include as special cases those examined by Keyfitz and
Kranzer and by Serre. They take the form
systems, and to a situation where, additionally, the condition of
genuine nonlinearity is violated throughout phase space. The systems
we consider include as special cases those examined by Keyfitz and
Kranzer and by Serre. They take the form
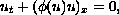
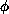 is a scalar-valued function of the n-dimensional
vector
is a scalar-valued function of the n-dimensional
vector
 , and
, and
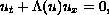
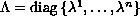 with
with
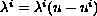 ,
where
,
where
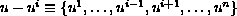 .
.